貝茲曲線:修訂版本之間的差異
出自六年制學程
(→一、) |
(→一、) |
||
| 第 17 行: | 第 17 行: | ||
<td>從目前點的座標畫條二次貝茲曲線到指定點的 x,y 座標:其中 x1,y1 為控制點</td> | <td>從目前點的座標畫條二次貝茲曲線到指定點的 x,y 座標:其中 x1,y1 為控制點</td> | ||
</tr></table> | </tr></table> | ||
| + | 二次貝茲曲線的參數方程為: | ||
| + | <span class="math math-inline"><span class="katex"><span class="katex-mathml"><math xmlns="http://www.w3.org/1998/Math/MathML"><semantics><mrow><mi>B</mi><mo stretchy="false">(</mo><mi>t</mi><mo stretchy="false">)</mo><mo>=</mo><mo stretchy="false">(</mo><mn>1</mn><mo>−</mo><mi>t</mi><msup><mo stretchy="false">)</mo><mn>2</mn></msup><mo>×</mo><mi>P</mi><mn>0</mn><mo>+</mo><mn>2</mn><mo stretchy="false">(</mo><mn>1</mn><mo>−</mo><mi>t</mi><mo stretchy="false">)</mo><mi>t</mi><mo>×</mo><mi>P</mi><mn>1</mn><mo>+</mo><msup><mi>t</mi><mn>2</mn></msup><mo>×</mo><mi>P</mi><mn>2</mn></mrow><annotation encoding="application/x-tex">B(t) = (1-t)^2 \times P0 + 2(1-t)t \times P1 + t^2 \times P2</annotation></semantics></math></span><span class="katex-html" aria-hidden="true"><span class="base"><span class="strut" style="height: 1em; vertical-align: -0.25em;"></span><span class="mord mathnormal" style="margin-right: 0.05017em;">B</span><span class="mopen">(</span><span class="mord mathnormal">t</span><span class="mclose">)</span><span class="mspace" style="margin-right: 0.2778em;"></span><span class="mrel">=</span><span class="mspace" style="margin-right: 0.2778em;"></span></span><span class="base"><span class="strut" style="height: 1em; vertical-align: -0.25em;"></span><span class="mopen">(</span><span class="mord">1</span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">−</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 1.0641em; vertical-align: -0.25em;"></span><span class="mord mathnormal">t</span><span class="mclose"><span class="mclose">)</span><span class="msupsub"><span class="vlist-t"><span class="vlist-r"><span class="vlist" style="height: 0.8141em;"><span style="top: -3.063em; margin-right: 0.05em;"><span class="pstrut" style="height: 2.7em;"></span><span class="sizing reset-size6 size3 mtight"><span class="mord mtight">2</span></span></span></span></span></span></span></span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">×</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 0.7667em; vertical-align: -0.0833em;"></span><span class="mord mathnormal" style="margin-right: 0.13889em;">P</span><span class="mord">0</span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">+</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 1em; vertical-align: -0.25em;"></span><span class="mord">2</span><span class="mopen">(</span><span class="mord">1</span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">−</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 1em; vertical-align: -0.25em;"></span><span class="mord mathnormal">t</span><span class="mclose">)</span><span class="mord mathnormal">t</span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">×</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 0.7667em; vertical-align: -0.0833em;"></span><span class="mord mathnormal" style="margin-right: 0.13889em;">P</span><span class="mord">1</span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">+</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 0.8974em; vertical-align: -0.0833em;"></span><span class="mord"><span class="mord mathnormal">t</span><span class="msupsub"><span class="vlist-t"><span class="vlist-r"><span class="vlist" style="height: 0.8141em;"><span style="top: -3.063em; margin-right: 0.05em;"><span class="pstrut" style="height: 2.7em;"></span><span class="sizing reset-size6 size3 mtight"><span class="mord mtight">2</span></span></span></span></span></span></span></span><span class="mspace" style="margin-right: 0.2222em;"></span><span class="mbin">×</span><span class="mspace" style="margin-right: 0.2222em;"></span></span><span class="base"><span class="strut" style="height: 0.6833em;"></span><span class="mord mathnormal" style="margin-right: 0.13889em;">P</span><span class="mord">2</span></span></span></span></span> | ||
==參考文章== | ==參考文章== | ||
*[https://zh.wikipedia.org/wiki/貝茲曲線 貝茲曲線] | *[https://zh.wikipedia.org/wiki/貝茲曲線 貝茲曲線] | ||
*[http://blog.iderzheng.com/continuous-and-smooth-bezier-curve/ 連續平滑的貝塞爾曲線] | *[http://blog.iderzheng.com/continuous-and-smooth-bezier-curve/ 連續平滑的貝塞爾曲線] | ||
2023年8月27日 (日) 13:49的修訂版本
二次貝茲曲線
- 二次貝茲曲線畫出的是拋物線,無法畫出橢圓和雙曲線。故無法畫出正圓。
- 所有拋物線都「相似」(不是相等),所有曲率的微線段都有。
- 兩端點外只有一個控制點。
- 拋物線方程式 ax2+bxy+cy2+dx+ey+f=0 則 b2 - 4ac=0 ,即前三項為完全平方式。
參考
- 二元二次方程式圖形判別的例題
---------- 擬合圓 ---------- - How to create circle with Bézier curves?
- 如何使用Bézier曲線創建圓?
- 用三阶贝塞尔曲线拟合圆
一、
| Q or q (quadratic Bézier curve) |
x1 y1 x y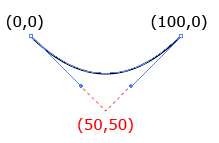 <path d='M0,0 Q50,50 100,0' style='stroke:black'/> <path d='m0,0 q50,50 100,0' style='stroke:black'/> |
從目前點的座標畫條二次貝茲曲線到指定點的 x,y 座標:其中 x1,y1 為控制點 | |
二次貝茲曲線的參數方程為: <math xmlns="http://www.w3.org/1998/Math/MathML"><semantics><mrow><mi>B</mi><mo stretchy="false">(</mo><mi>t</mi><mo stretchy="false">)</mo><mo>=</mo><mo stretchy="false">(</mo><mn>1</mn><mo>−</mo><mi>t</mi><msup><mo stretchy="false">)</mo><mn>2</mn></msup><mo>×</mo><mi>P</mi><mn>0</mn><mo>+</mo><mn>2</mn><mo stretchy="false">(</mo><mn>1</mn><mo>−</mo><mi>t</mi><mo stretchy="false">)</mo><mi>t</mi><mo>×</mo><mi>P</mi><mn>1</mn><mo>+</mo><msup><mi>t</mi><mn>2</mn></msup><mo>×</mo><mi>P</mi><mn>2</mn></mrow><annotation encoding="application/x-tex">B(t) = (1-t)^2 \times P0 + 2(1-t)t \times P1 + t^2 \times P2</annotation></semantics></math>B(t)=(1−t)2×P0+2(1−t)t×P1+t2×P2